Spin-orbit coupled Hamiltonian

For applications in many parts of condensed matter physics and cold atoms physics, we use what is known as the Rashba spin-orbit coupled Hamiltonian. This Hamiltoninan is so-named because it couples momentum $\mathbf{p}$ to the spin $\mathbf{S}=\frac{1}{2}\sigma$ where $\sigma =({\sigma }_x,{\sigma }_y,{\sigma }_z)$ are the Pauli matrices and $\mathbf{p}=(p_x,p_y,p_z)$ is a vector of momentum operators:

$$\begin{array}{}\text{(1)}& H=\frac{p^2}{2m}+\alpha (\mathit{\sigma }\times \mathbf{p})\cdot \hat{\mathbf{z}}+\mathrm{\Delta }{\sigma }_z.\end{array}$$

$m$ is the mass, $\alpha$ is the spin-orbit coupling strength, and $\mathrm{\Delta }$ is some Zeeman field (it acts as magnetic field on the spin).

In this post, we go through the calculation of the energy spectrum and eigenvectors – a straight forward exercise in undergraduate linear algebra.

First of all, instead of the normal method of finding eigenvectors, we note that we can rewrite this Hamiltonian in the form

$$\begin{array}{}\text{(2)}& H=\frac{p^2}{2m}+\mathbf{b}(p)\cdot \mathit{\sigma }\end{array}$$

where $\mathbf{b}(p)=(\alpha p_y,-\alpha p_x,\mathrm{\Delta })$. Now, $\mathbf{b}(p)$ represents a point on the Bloch sphere, and so we expect the eigenvectors to be parallel and anti-parallel to this vector. The energies in this case are very straight forward and amount to the positive and negative of $|\mathbf{b}(p)|$:

$$\begin{array}{}\text{(3)}& {ϵ}_{\pm }(p)=\frac{p^2}{2m}\pm \sqrt{{\alpha }^2{p}^2+{\mathrm{\Delta }}^2}.\end{array}$$

With these eigenvalues, it is a straight forward exercise in linear algebra to find the eigenvectors. After a bit of algebra, the eigenvectors of $H$ in terms of the eigenvectors of ${\sigma }_z$ ( ${\sigma }_z|\uparrow ⟩=|\uparrow ⟩$ and ${\sigma }_z|\uparrow ⟩=-|\uparrow ⟩$ ) are

$$\begin{array}{}\text{(4)}& |\pm ⟩=\frac{1}{\sqrt{2}}[\sqrt{1\pm \frac{\mathrm{\Delta }}{\sqrt{{\mathrm{\Delta }}^2+{\alpha }^2{p}^2}}}|\uparrow ⟩+e^{-i\varphi }\sqrt{1\mp \frac{\mathrm{\Delta }}{\sqrt{{\mathrm{\Delta }}^2+{\alpha }^2{p}^2}}}|\downarrow ⟩]\end{array}$$

where we have defined $\varphi$ by $p_y+i{p}_x=p{e}^{i\varphi }$. Note that when $p_{x,y}\to -p_{x,y}$, the occupations stay the same. However, if we just look at one energy, ${ϵ}_{-}(p)$ the ground state energy, we see that the state we get when $p_{x,y}\to -p_{x,y}$ is almost orthogonal to the original state.

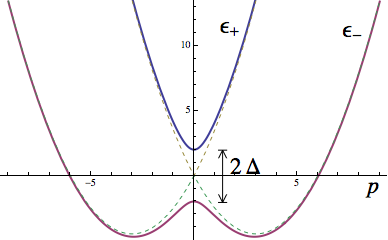
The energy bands themselves look like the figure on the right where the vertical axis is energy (and for this particular example, $m=1$, $\alpha =3$, and $\mathrm{\Delta }=2$). Interestingly, the introduction of $\mathrm{\Delta }$ actually causes the gap to open up – the dotted lines are for when $\mathrm{\Delta }=0$.

Now, if we have a bunch of fermions filling up these energies, if we set the chemical potential to be in the gap, we would find that the only excitations would states that are spin-locked to the momentum.

Many things can be done with this Hamiltonian to interesting effect. It finds its way into cold atom physics as well as condensed matter.