An image quilt of quasicrystals

Quasicrystals, a beautiful manifestation of something without a strict crystalline symmetry but nonetheless shows order, have won a Nobel prize and have recently interested my own work with a dodecagonal graphene quasicrystal making its way into Science1.

This led to this beautiful cover in Science2

Cover image from a Science magazine cover story.

This phenomena is a perfect example of the kind of research I’ve been doing a lot with these days, and so it inspired the new logo for this website

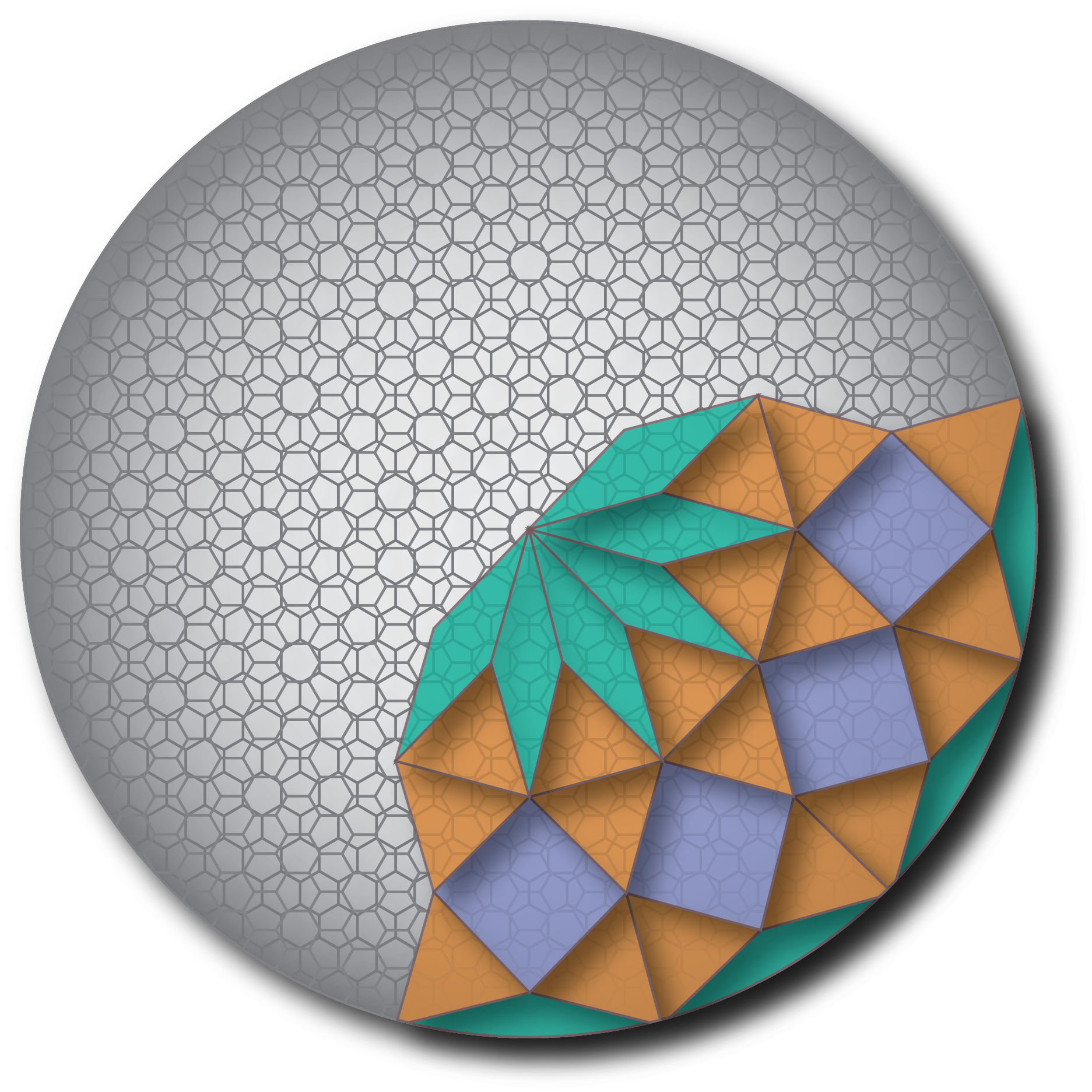
Building a Penrose tiling from two sheets of graphene twisted at 30-degrees with respect to each other.

One can tell how this is done: You find the points where two hexagons are on top of each other, put down a point, and connect. There are three shapes: a rhombus, an equilateral triangle, and a square. This can be done along the entire sheet to create an amazing looking pattern. For completeness, we can fill in the rest of the pictured grid to obtain:

A fully Penrose tiled sheet.

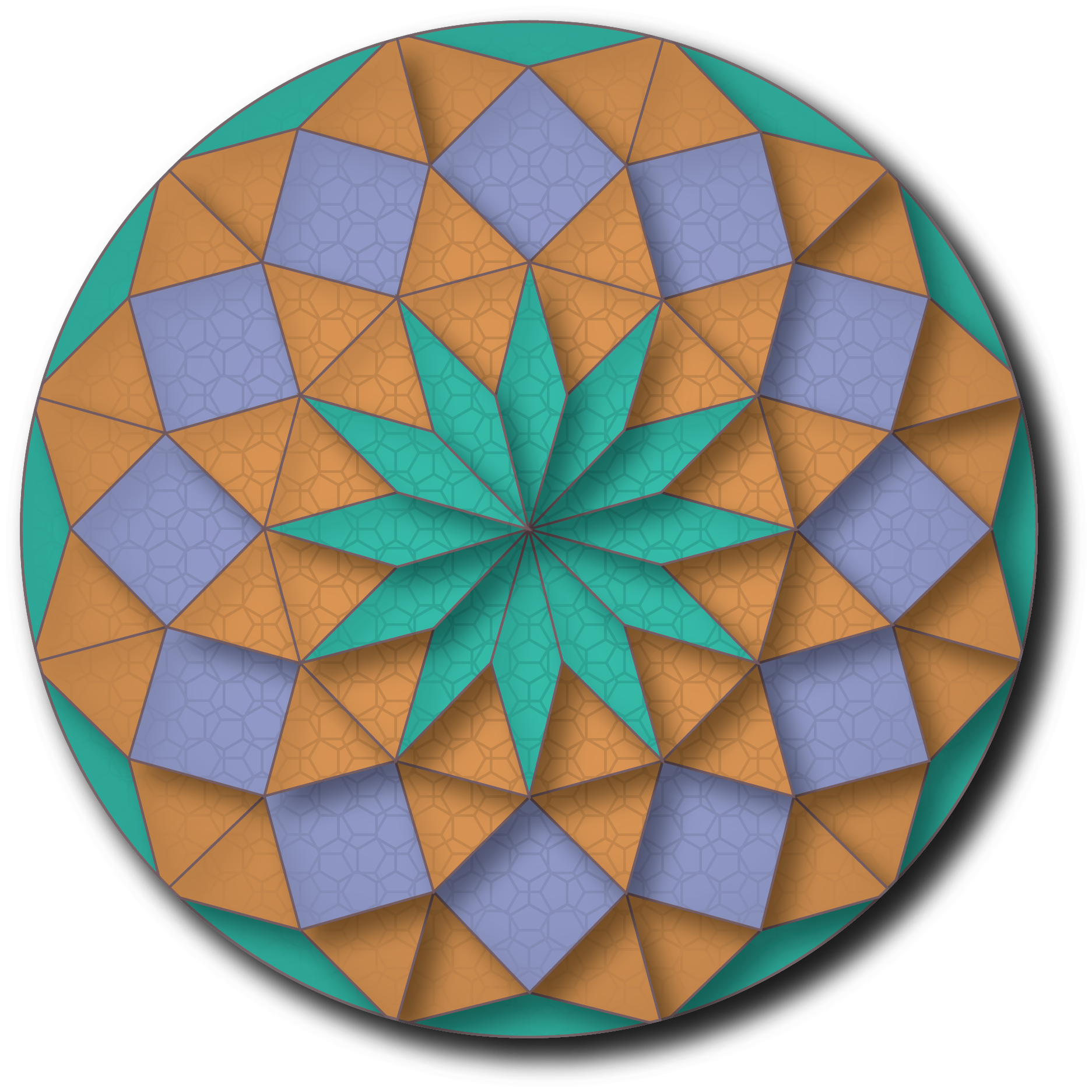
The pattern starts to look even more intriguing the further out in the tiling you go. There is much to learn about such physical systems and their quasiperiodic cousins.

Footnotes

  1. S. J. Ahn et al., Science 361, 782 (2018)’ ↩

  2. We have been studying how quasiperiodicity interplays with materials that have Dirac nodes, including twisted bilayer graphene. While we have not studied graphene at 30-degrees like the work in Science, that is an extreme where all crystalline periodicity is lost. ↩